PROBLEM DESCRIPTION: Linked List Cycle - Find Start of Cycle
If there is a cycle in the LinkedList, find the Node where the cycle starts.
SOLUTION
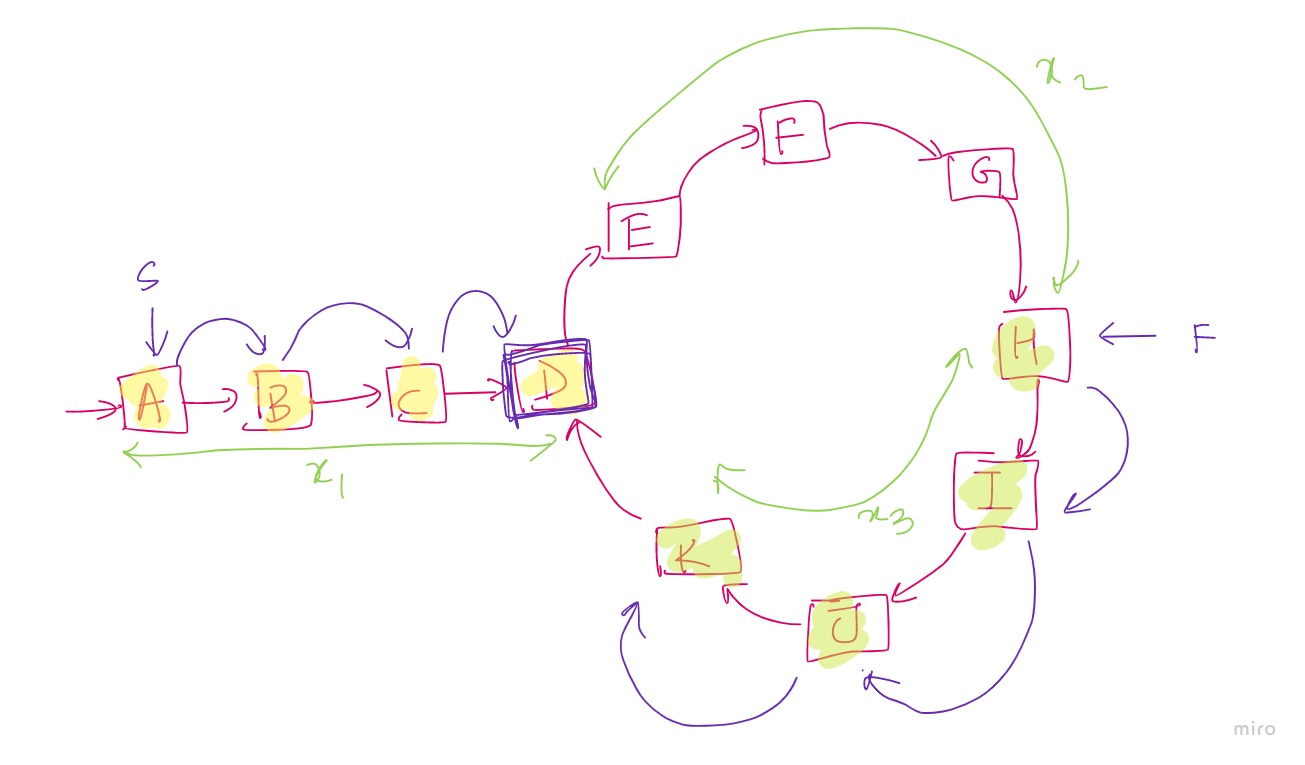
We can easily find if the cycle exists by using slow and fast pointers. If they meet, we know that there is a cycle in the list. From the intersection point in the cycle, we need to do the following:
- Move fast node by 1
- Reset slow node to head
- Move both fast and slow one step at a time until both are same
- Return the node which is same (entrypoint of the cycle)
Intuition
Let’s say:
x1 = length from start to endtrypoint of cycle
x2 = length from entrypoint to intersection of slow and fast
x3 = length from intersection of slow and fast to entrypoint
[Refer to the above picture]
We can say that:
The length travelled by fast is 2 times the length travelled by slow.
=> x1 + x2 + x3 + x2 = 2(x1+x2) -» Important note: This is when ignoring multiple cycles by fast in certain scenarios
=> x1 = x3
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
public class Solution {
public ListNode detectCycle(ListNode head) {
if(head == null) return null;
ListNode slow = head;
ListNode fast = head.next;
while(fast != null && fast.next != null){
slow = slow.next;
fast = fast.next.next;
//If slow==fast, cycle exists. We have found the intersection point.
if(slow == fast){
//We move the fast node one step further and reset the slow node to head. Then we keep moving them one step at a time until they meet. This will be our answer - start Node of the cycle
fast = fast.next;
slow = head;
while(slow != fast){
slow = slow.next;
fast = fast.next;
}
return slow;
}
}
return null;
}
}
